Bridges Towson 2012

Bridges Towson 2012
FAMILY DAY & MATH-ART EXPO
Coordinated by Kristóf Fenyvesi, Director of Community Events, Bridges Organization
E-mail: familyday@bridgesmathart.org, Phone: +358-45-256-0420

In 2012, the Bridges Conference, the largest mathematics and art interdisciplinary conference in the world was held at the Towson University,  Baltimore. On 28 July, 2012, we opened the gates of Towson University, Center for the Arts wider than ever and invited everyone of all ages to browse among our rich collection of artistic and scientific workshops and fun programs.

WE OFFERED…

1. Workshops For Families and Bridges Participants
2. Short Movie Festival
3. Experimental Theater
4. An Afternoon of Mathematical Poetry
5. Mime-Matics Night
6. Informal Music Event
7. Bridges Of The World! Jardin Galerie’s Children and Youth Art Exhibit

1. Workshops For Families and Bridges Participants

Teaching Temari: Geometrically Embroidered Spheres in the Classroom
by Carolyn Yackel (Mercer University)
This workshop introduced the various potential pedagogical benefits of using temari balls, or geometrically embroidered spheres in a mathematics class, either to craft or as objects of investigation. During this intensive workshop, participants simultaneously learned to construct temari balls and discussed ways to utilize temari balls to enhance student understanding of a variety of mathematical concepts.

 

Creating Non-Systematic Islamic Geometric Patterns With Complex Combinations of Star Forms
by Jay Bonner (Bonner Design Consultancy)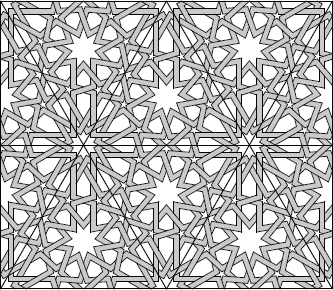
This workshop demonstrated the design methodology employed in the creation of particularly complex Islamic non-systematic geometric patterns with differentiated regions of compound local symmetry. This variety of Islamic geometric pattern is characterized by combinations of star forms that range from the more compatible
(such as 9s and 12s) to the seemingly incompatible (such as 9s and 11s, and 11s and 13s). A series of historical pattern examples, ranging in complexity, were used to demonstrate the non-systematic use of the Polygonal Technique of geometric pattern generation; and corroborating historical evidence was provided that confirms that the techniques being taught in this Workshop were used by Muslim artists of the past. Along with self-similar designs, this variety of geometric pattern represents the pinnacle of Islamic geometric art; yet very little has been published on this all-but-lost design methodology. The objective of this Workshop was to assist in the rekindling of this design tradition: opening the door to working with, and teaching, the more complex aspects of this ancient design discipline.

A Workshop in the Persian Decorative Art of Tazhib
by Mojgan Lisar
Tazhib (Illumination) is a classical Persian art for the decoration of treatise and books. It has an intertwining relationship with calligraphy. In the medieval Persia, a highly refined art of Tazhib developed that its tradition has continued even today. In a traditional Persian Tazhib one can find mathematical ideas and concepts such as symmetries, logarithm and Archimedean spirals, polygons and star polygons. We used the traditional methods used in this art to create a Tazhib symbol.

Let’s Make a Chiral Tessellation Dance
by Joseph D. Clinton
This workshop gave educators and their students’ hands-on experience to understand the differences between theoretical abstraction and the reality of applying physical restraints. Each participant in the workshop received a modeling kit to assemble and take with her/him. The model illustrated the physical realities of applying the mathematical principles of rotation and translation transformations of a linkage of triangles from one symmetry form to another. Each participant also received an animated film that illustrate the theoretical application of the same mathematical abstractions.

Kolam Workshop
by Shanthi Chandrasekar

Indian women often begin their day and sometimes also end it by drawing kolams on the ground just outside the front door. These repeating patterns, a type of Tantric Art, have been passed down from generation to generation for centuries, and symbolize the scientific and philosophical patterns innate to and infinite throughout the cosmos. Like Native American sand paintings or Buddhist mandalas, the kolams are part of the cycle of creation and destruction. The Kolam workshop  included an introduction to the art form and its mathematical significance. The participants learned to draw basic Kolam drawings during the workshop.

 

Bead Crochet Bracelets: What Would Escher Do?
by Ellie Baker and Susan Goldstine (Department of Mathematics and Computer Science, St. Mary’s College of Maryland)
In this workshop, we presented bead crochet bracelets that we have designed in the style of M.C. Escher’s tessellations using a powerful new technique for extracting bracelet patterns from planar tilings. Since the craft of bead crochet is too intricate to master in one short session, we taught participants the bead crochet stitch with large plastic beads and yarn, and allowed them to create their own bead crochet patterns consisting of interlocking copies of a single shape in different colors. Participants also received the materials for a simple bead crochet bracelet in smaller glass seed beads so that they could practice real bracelet making after the workshop.

Jardin Galerie’s Bridges Workshop:Modelability & Minimum Systems
by John Hiigli (Jardin Children’s Art Galerie, New York City), Stephen Metcalf, Stephen Taylor
John Hiigli’s presentation on the Synergetic Block System, followed by a demonstration of the „simplest allspace-filler” of Synergetic Geometry: the Mite. Finally children were given the opportunity to build with Hiigli’s extensive block system of more than 576 blocks. The goal was to provide opportunities for omni-directional modeling within a system characterized by simple integral volume ratios of common solids: tetrahedron, octahedron, rhombic dodecahedron, cube octahedron, etc.

 

Stephen Metcalf, who is a sculptor from Providence, Rhode Island invited the children to build 6 strut tensegrities and assemble them into a chain that can be arched into a bridge.

 

Rhythm Necklaces: Workshop in Music and Mathematics
by Stephen Taylor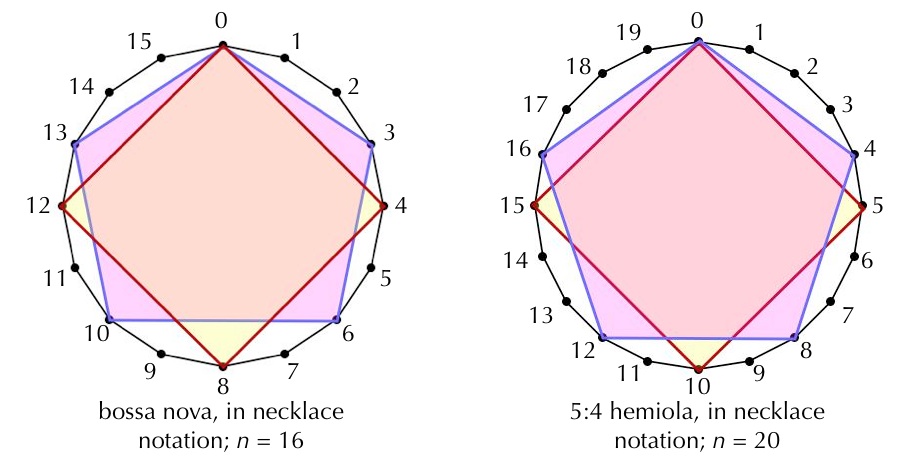
This workshop introduced the concept of rhythm necklaces – rhythms which can be represented as polygons on a circle. These necklaces helped participants to learn about musical and mathematical concepts, including hemiola and maximal evenness, while also learning several African and Cuban syncopated rhythms.

Math and Dance – Windmills and Tilings and Things
by Karl Schaffer (Co-Artistic Director Dr. Schaffer and Mr. Stern
Dance Ensemble and De Anza College)
This workshop explored integrating mathematics and dance in the classroom as well as on stage. We involved several mathematical topics which were embodied and explored by participants. These included play with circular motions, use of paper as a prop, video and rhythmic tessellations and patterns, and N-body choreographies. Participants created, practiced, and performed short dance phrases, and simultaneously explored mathematical principles and critique the work from the point of view of both the mathematics and the artistry involved.

Explore, Improvise, Construct, Dance! /in 3 sessions/
by Karen Kuebler (Arts Integration Specialist; Towson University – Baltimore County Public Schools)

Participants actively engaged in math, art, and movement. They also had the opportunity to use a variety of props that encourage learning about shapes (2D and 3D) and structures in the environment.

Activity 1: Connecting Shapes
Participants received a variety of shape props in order to explore the attributes of these six basic shapes. Then, I led a structured improvisation that classified and connected the shapes according to their attributes. The activity culminated in an entire group connected shape!

Activity 2: 3D Shape Exploration and Construction
Participants explored building structures with a variety of 3D blocks in small groups. Participants discussed shapes and how they fit together to form structures. The “pièce de résistance” was the opportunity to knock the structure down and start again with a different group of 3D blocks.

Activity 3: Monet’s Waterlilies and Pond
After viewing a short slideshow from Monet’s Home and Garden in Giverny, France, participants did a guided improvisation of the life cycle of a flower from seed to bloom. Then, participants were led through a structured improvisation of Monet’s pond and created the Japanese footbridge sculpture. In between the sessions, Karen were running different slideshows showing students doing the above activities.

Digital Sangaku Workshop
by Jean Constant and Radmila Sazdanovic
Theme: The arbelos. – Solve the math problem / Explore digital visualization techniques / Create your own Digital Sangaku. Sangaku were classic mathematical problems etched or painted on wooden tablets during the Edo period in Japan. Radmila Sazdanovic guided the attendees through the mathematical issue at hand. Jean Constant invited them to revisit the original visualization and create their own interpretation using available computer aided graphic editing tools and modern visualization techniques. This workshop followed Jean Constant short lecture and exhibition of 3 original digital Sangaku visualization at the occasion of the conference. More info on Sangaku at http://www.hermay.org/jconstant/wasan/
Radmila Sazdanovic is a Postdoctoral Researcher in the Department of Mathematics, University of Pennsylvania. Her mathematical research interests include knots, links and their invariants, categorification of combinatorial structures, and applied topology. Jean Constant taught digital media as Acting Director of the Visual Communication Program for the Mathematics and Engineering Department of the Northern New Mexico College and is now is Consultant in Media Technology.

Creating Lovely Islamic Geometric Patterns With Islamic Geometric System Tiles
by Jay Bonner, Amina Buhler-Allen, Marc Pelletier
Repetitive polygonal tessellating elements were utilized as a means to create Islamic geometric patterns from as early as the tenth century. Over time, Muslim artists devised several symmetrical repetitive systems that prove highly versatile in creating a wide diversity of very beautiful geometric designs in each of the four principle pattern types: acute, median, obtuse and 2-point. Since first bringing to market these sets of these Islamic Geometric System Tiles in 2000, the authors have collaborated to create a comprehensive series of sets comprised of magnetic tiles, with printed pattern lines in each of the various pattern families. Participants in this Workshop had the opportunity to create original geometric patterns from a wide selection of these sets. Children and adults alike were welcome.

Zometool Play
by Paul Hildebrandt (co-inventor and current president of Zometool)
It was a fun way for kids (and parents) to get their hands on (and wrap their minds around) a powerful mathematical tool while building big, beautiful and altogether amazing structures from 2, 3 and higher dimensions! Do let Zometool’s brightly colored, shape-coded components fool you: even though several Nobel prizewinners “play” with Zometool (including 2011 Chemistry winner Daniel Schechtman), the point of Zometool is to have fun — the real kind of fun that we artists and mathematicians live for — learning, discovery and creation. Zometool co-inventor and current President Paul Hildebrandt facilitated this “playshop,” i.e., give gentle guidance while trying to keep all hell from breaking loose.

Making Tessellated Polyhedra
by Robert Fathauer

 

Polyhedra nets printed on card stock with Escher-like designs were available to color, cut out and score, and tape or glue to form polyhedra.

 

 

Quilt Fun With √2
by Elaine Krajenke Ellison

Each participant was given a two-sided tag board pattern. The mathematics of the quilt pattern was shown on one side of the tag board. The second side had a quilt pattern that has no color. The participant cut colored paper or sticky felt paper patterns. The colored paper was attached to the blank template provided. A beautiful mathematical design was created.

 

 

Exhibit of Beautiful Icosahedra
by Eve Torrence (Randolph-Macon College)

Eve Torrence displays the paper models that can be created from the pages of her new book: “Cut Assemble Icosahedra: Twelve Models in White and Color”. The models were colored in a way that revealed some beautiful geometry. This geometry is interesting to professional mathematicians and yet can also be understood by children.

 

Countless challenges to brainy builders with JOVO
supported by the JOVO International
by Kristóf Fenyvesi (Experience Workshop, University of Jyväskylä)
JOVO is a widespread and recognized construction toy that holds a multiple set of educational benefits together with play. It is also a resource for teaching Maths, 2 & 3 dimensional geometry, technology and design. JOVO is not based on specific set of constructions as its purpose is to inspire and not to predestine its use. JOVO continously challenges childrens imagination, creativity and inventiveness. JOVO tries to provoke the children to find new ways and solutions.

SAXON’s PolyUniverse Toys
supported by the Poly-Universe Ltd.
You may arrange the colored plastic sheets of the PolyUniverse artistic game on the table as you like them. A bigger colored figure appears in front of you when you put all the sheets down. There are various forms at the corner of the sheets with different colors and sizes. Depending on how you placed the sheets, these forms will show different figures. There will be symmetric figures, and also chaotic ones. It’s possible that some will resemble even flowers or crystals! Millions of variations and it depends on your imagination how the final picture will look like!

Family Day Ice Cream Social!

 

We celebrated the beauty of math under the BigZome sculpture at the close of Bridges Family Day. 

 

…AND!!!

 

 

Juggler, mathematician, and former circus performer Mike Naylor made random appearances with 10-minute mathematical juggling performances that demonstrated how mathematics can be found in artistic activities, and how mathematics can be used to create art.

 

 

 

2. Short Movie Festival

The 3rd Annual Bridges Short Movie Festival included a variety of juried and curated videos and short films. The program included movies, videos and animations that have been created for educational, corporate and artistic purposes, and provided another opportunity for you to experience innovative and integrative techniques in the fields of mathematics and art.

Coordinators: Amy and Nathan Selikoff

3. Experimental Theater: Albert’s Bridge by Tom Stoppard

The Bridges Community Players  Presented:  Albert’s Bridge by Tom Stoppard
Regarded as one of the leading playwrights of our time, Tom Stoppard was from the beginning interested in incorporating mathematical and scientific ideas into his creative work.  Twenty-five years before Arcadia, and just before Rosencrantz and Guildenstern Are Dead launched Stoppard into the limelight, the self-educated writer of ideas penned this fascinating one act tragic-comedy for radio. With their trademark verve and panache, the Bridges tragedians will bring Albert and his fellow bridge painters to life – not simply as a radio play, but in their full three dimensional glory.

Directed by Steve Abbott.

4. An Afternoon of Mathematical Poetry

“Newton’s binomial is as beautiful as Venus de Milo. What happens is that few people notice it.” (Fernando Pessoa [as Álvaro de Campos], translated from the Portuguese by Francisco Craveiro)

Representing a range of mathematical poetry from traditional to multimedia and from lyrical to visual, ten poets read selections from their work: Stephanie Strickland, Kaz Maslanka, Alice Major, Geof Huth, Philip Holmes, JoAnne Growney, Emily Grosholz, Marion Deutsche Cohen, Tatiana Bonch-Osmolovskaya and Sarah Glaz

Open microphone reading: There was an open microphone period at the end of the reading where Bridges participants read their mathematical poems.

Coordinator: Sarah Glaz (University of Connecticut)

5. Mime-Matics Night

Tim and Tanya Chartier’s mime combines masks, puppetry, and classical mime illusions into a distinctive style that they have performed throughout the United States and in national and international settings. The Chartiers have trained at Le Centre du Silence mime school, the Dell’Arte School of International Physical Theater and with the world-renowned mime artist Marcel Marceau. Dr. Tim Chartier is a professor of mathematics at Davidson College, North Carolina, USA. His ability to communicate math both in the classroom and through mime to the broader community. Tanya Chartier has taught theater at the middle school level and is currently an Educational Specialist for a center for academic learning in the town of Davidson and taught in the spring of 2012 in the Education Department at Davidson College. Playing with different sets of tiles related to the pentagonal family of traditional geometric patterns, and to contemporary mathematics as well.

6. Informal Music Event

Music is always a part of every Bridges conference. As one can see from the titles of the pervious proceedings books there are always a large number of contributed sessions given over to the intricacies of music, musical styles, and rhythm with mathematics. The conference participants who are music performers, presented a night of music and educated the audience about their new findings in performing music and its connections with mathematics.

Coordinator: Vi Hart

7. Bridges Of The World!
Jardin Galerie’s Children and Youth Art Exhibit
at Towson University‘s Center for the Arts
Curated by
John Arden Hiigli, founder-president of Jardin Galerie
&
Kristóf Fenyvesi, Director of Community Events, Bridges Organization

We organized a special “Children & Youth Exhibit” Bridges of the World in Baltimore, Maryland at Towson University’s Center for the Arts. It was a screened exhibition at Towson as part of the Family Day Celebration for students and young artists.

In addition there was an online exhibition at Jardin Galerie’s Facebook page.

Artists ages 4-18 years were invited to submit for exhibition a drawing or painting of a real bridge, drawn or painted en plein air. Submitted artwork were judged by a jury composed of representatives of Bridges and of Jardin Galerie. Prizes were awarded at Bridges 2012 for the “top three” works of art and the “best bridge” of the ”Children & Youth Exhibit.”

More Information on the Exhibit (click)

 

 

 

As always, admission was FREE to ALL EVENTS of the Bridges Family Day!

Questions, comments & more information:
Kristóf Fenyvesi, Director of Community Events, Bridges Organization
E-mail: familyday@bridgesmathart.org, Phone: +358-45-256-0420